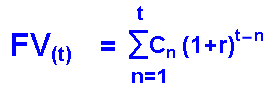Future Value with Uneven Cash Flows – Ordinary future value
Future value with uneven cash flows will be received from different uneven cash flows which are deposited or invested with appropriate discount rate during more than one period.
The ordinary cash flows occur at the end of each period.
Time Line
FV(t)= C1*(1+r)t-1 + C2*(1+r)t-2 + C3*(1+r)t-3 + C4*(1+r)t-4 + C5*(1+r)t-5 + …..+ Ct-1*(1+r)1 + Ct
Which:
- FV : future value
- Cn(n=1,2,3,4,…,t) : present value , principal amount or amount paid for the end of each period.
- r : interest rate, rate of return, or discount rate per period- typically, but not always, one year.
- t : number of periods- typically, but not always, the number of years.
- (1+r)t called future value interest factor or future value factor for $1 invested at r percent for t periods .
Question
Mr. BB deposits $300, $400,$500 and $700 in the bank for January, February, March and April respectively with interest rate of 2 % per month. How much money will Mr.BB has in the next 4 months? And Find interest? ( assume Mr.BB deposits at the end of each month.)
Solution
Time line
FV =300(1+0.02)3 + 400(1+0.02)2 + 500(1+0.02)1 + 700
= 300*1.0612 + 400*1.0404 + 500*1.0200 +700
= 318.36+416.16+510+700=$1,944.52
This means that in the 4 months Mr.BB will have $ 1,944.52
And Interest = FV – each amount paid= $ 1,944.52-(300+400+500+700)=$44.52